Tyrannosaurus rex, Osborn, 1905

Rayfield, Emily J., 2004, Cranial mechanics and feeding in Tyrannosaurus rex, Proceedings of the Royal Society of London, Series B 271 (1547), pp. 1451-1459 : 1452-1458

2 . MATERIAL AND METHODS

Specimens studied: AMNH 5027: American Museum of Natural History, New York ; BHM 3033: Black Hills Museum, Hill City, South Dakota ; MOR 555: Museum of the Rockies, Bozeman, Montana ; SDSM 12047: South Dakota School of Mines, Rapid City, South Dakota ; RTMP 81.6.1: Royal Tyrell Museum of Palaeontology, Drumheller, Canada .

(a) Anatomical observations of sutural mobility

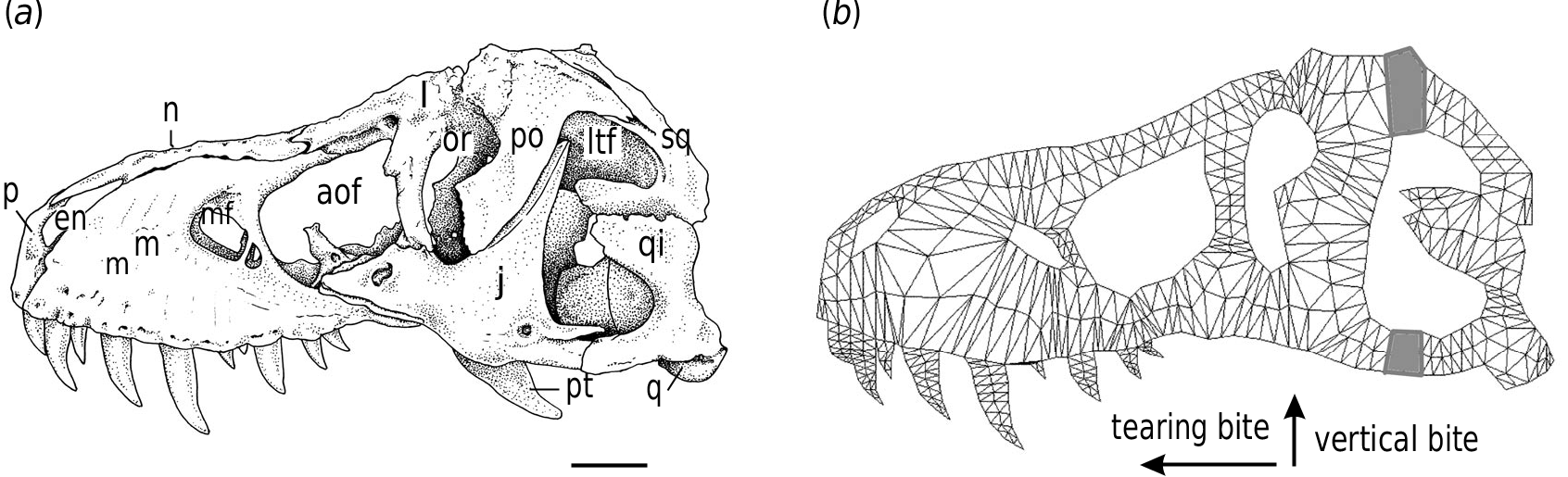
Four facial sutures commonly appear patent and slightly mobile in T. rex skulls observed. These are the maxilla–jugal, postorbital–jugal, quadratojugal–jugal and postorbital–squam-osal contacts. Two of these sutures are not universally mobile: the quadratojugal–jugal suture is fused in some specimens (e.g. AMNH 5027 ) and movement at the postorbital–squamosal would be restricted by attachment of the superficial and possibly medial slips of the M. adductor mandibulae externus, which originate in part along the lateral supra-temporal fenestra margin. The remaining two sutures, namely the maxilla–jugal and postorbital–jugal contacts, remain patent in nearly all observed specimens, and are the main focus of this analysis. Patent, yet apparently immobile, sutures exist between many other cranial bones, and future analysis will attempt to elucidate the significance of these sutures. It should be noted that although the FEMs use a particularly loosely articulated skull ( BHM 3033 ; figure 1 ) as a template, the following descriptions of cranial mobility are based on observations of numerous specimens (see above).

(b) Jugal–postorbital contact

The postorbital laps a smooth groove running down half the length of the anterior surface of the ascending process of the jugal ( figure 2 a ). Postorbital–jugal contact surfaces are variably rugose, with BHM 3033 bearing smooth articulation surfaces while ANMH 5027 and MOR 555 possess more rugose surfaces along the length of the contact. Furthermore, in AMNH 5027 and MOR 555 , the anterior surface of the lower half of the ascending process bears a pronounced roughened region that marks the ventral extent of postorbital overlap. A depressed groove running along the posterior surface of the descending process of the postorbital marks the contact with the jugal. In all specimens observed and those documented in the literature (e.g. Brochu 2003) this contact is patent and potentially mobile, with the exception of MOR 008, in which the left jugalpostorbital contact is fused internally, probably as a result of the advanced age of this specimen ( Molnar 1991). Additionally, minor interdigitations at the anterior edge of the postorbitaljugal suture in AMNH 5027 may have limited movement along the suture in this particular skull. Overlapping flanges at the postorbital–jugal contact surface generally prevent rotation in the transverse and parasagittal axis, but sliding of the jugal anteroventrally–posterodorsally against the postorbital is permitted ( figure 2 a ).

(c) The maxilla–jugal contact

The anterior portion of the jugal forks medially and laterally, ventral to its contact with the lacrimal. The medial fork laps on to the medial surface of the maxilla while the lateral fork further divides into a dorsal and ventral component, between which slots a narrow process of the maxilla (as noted by Molnar (1991)). Additionally the dorsal edge of an extended maxillary process laps the ventrolateral edge of the jugal along a posteriorly extended groove ( figure 2 b ). In none of the observed specimens was the maxilla–jugal contact fused. Dorsoventral and mediolateral movement plus rotation about the transverse and parasagittal axes is prevented by the interlocking mediolateral and dorsoventral articulations. The distinct anteroposterior orientation of all contacts suggest that slight anteroposterior sliding movement plus some limited rotation about the longitudinal axis of the jugal is permitted at this suture ( figure 2 b ).

(d) Finite element modelling

A two-dimensional (2D) FEM of a T. rex skull was created. A lateral-aspect photograph of BHM 3033 (Hell Creek Formation, South Dakota; figure 1 a ) was digitized in SCION IMAGE (www.scioncorp.com). Outline x, y coordinates were imported into the Geostar geometry creator component of the COSMOSM FEA package (v. 2.0 for Unix; SRAC Corp. CA, USA and Cenit Ltd, UK). A series of 5 cm thick surfaces was created then ‘meshed’ to produce an interconnected grid of three-noded triangular FEs representing the lateral aspect of the cranium ( figure 1 b ). Each element was attributed the mechanical properties of bovine Haversian bone after Rayfield et al. (2001).

The model represents a 2D section of the left aspect of the skull: the palate and braincase were not included. 2D models are used as a first approximation in orthopaedic biomechanical modelling, and using simple FEMs offers the potential to generate mechano-functional hypotheses ( Carter et al. 1998), which may be further tested by digitally modifying future models. The 2D models presented here were constrained from moving about the lower temporal fenestra ( figure 1 b ) to focus upon the stress response of the rostrum, which as a more planar structure than the posterior skull is more appropriate for 2D modelling. Stress patterns posterior to the constraining surfaces, including the effect of condylar and muscular forces in the posterior skull, were not analysed and this region of the skull should therefore be ignored in relevant figures.

Four structurally different FEMs were constructed by manipulating the base model: an initial ‘fused’ solid model with no mobile regions ( figure 1 b ) and three modified ‘mobile’ models showing differing degrees of intracranial mobility; a mobile post-orbital–jugal suture ( figure 2 c ), a mobile maxilla–jugal suture ( figure 2 d ), and a model with both a mobile maxilla–jugal and postorbital–jugal suture (not shown). The mobile FEMs ( figure 2 c, d ) were created by introducing breaks in the FE-mesh at the location of the appropriate suture in the actual skull.

(e) Bite force magnitude and distribution

Tyrannosaurus rex may have been capable of generating 13 400 N bite force at a single posterior tooth ( Erickson et al. 1996). Using moment arm calculations to extrapolate this value rostrally along the tooth row, a total of 78 060 N was divided between biting teeth (therefore assuming 156 120 N bilaterally, less than, but approaching, values estimated by Meers (2002)). However, it may be argued that being first to contact a prey item, the large caniniform teeth received the majority of bite force (sensu Rayfield et al. 2001). In accordance with this suggestion, the two large caniniform teeth ( figure 1 b ) were allocated 13 000 N each, while the smaller incisiform and posterior maxillary teeth were allocated lesser values scaled to the size of the teeth. In this model a total of 31 000 N was applied.

FEAs were performed to assess the stress response to this load in a fused or mobile skull. First, vertical dorsally directed bite forces representing the ‘puncture’ aspect of feeding were applied to the tooth tips in all four models and the corresponding stress and strain patterns were calculated. The analyses were then rerun applying instead a horizontally orientated, anteriorly directed bite force to represent the ‘pull’ tearing force, generated by the resistance of flesh and bone against the teeth during tugging and flesh-procuring behaviour ( figure 1 b ). Multiple tearing analyses applying moment-calculated forces, variable tooth-sizerelated forces and equal forces to all teeth were investigated. Because bite force was hypothetical but identical in related models, relative rather than absolute patterns of stress and strain could be assessed.

3. RESULTS

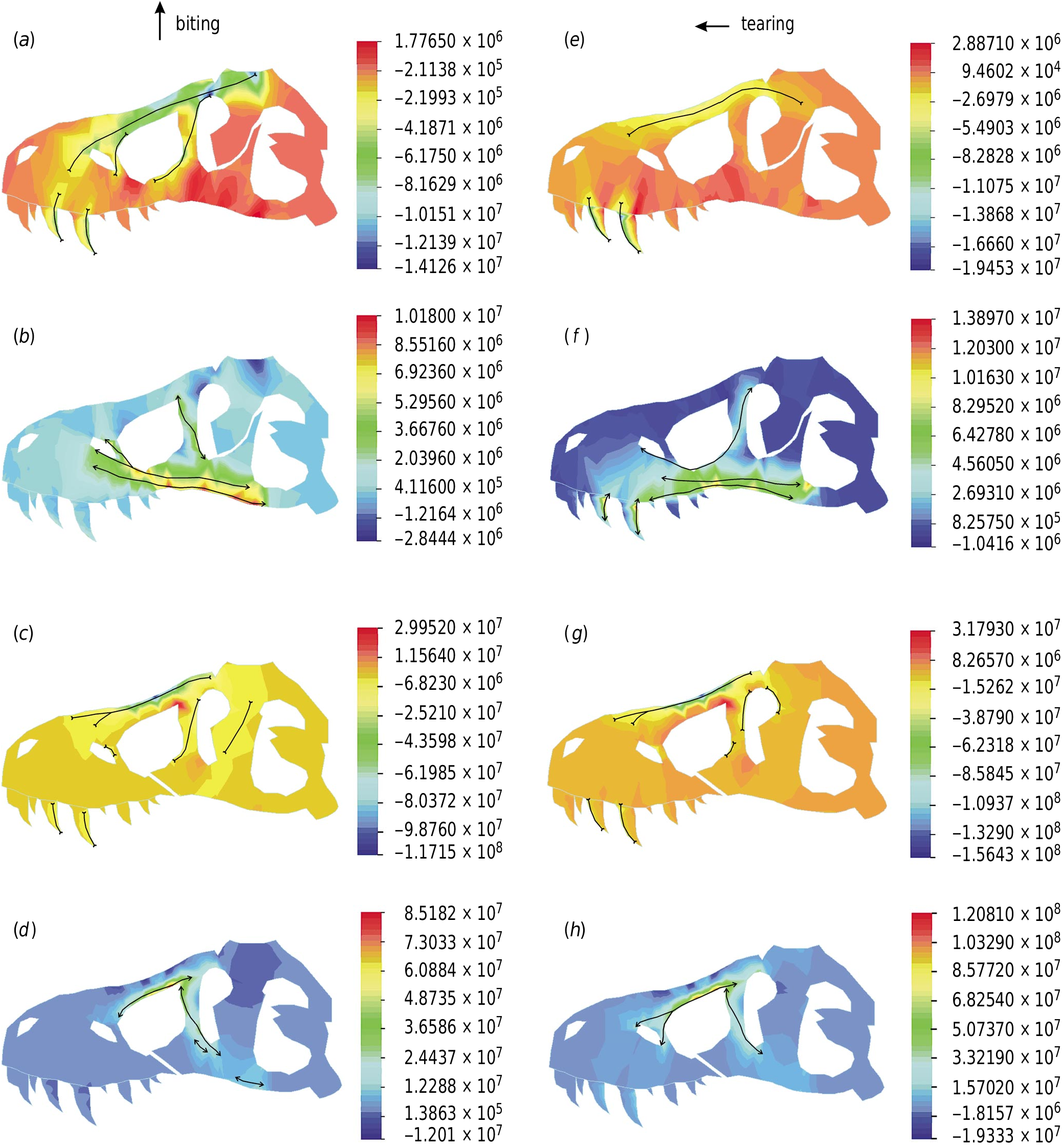
Colour-coded stress distribution plots with superimposed stress vector orientation illustrate the pattern of stress and strain in the skull under biting and tearing loads ( figures 3 and 4 and electronic Appendices A–C). By convention, tensile stresses and strains are allocated positive values, whereas compressive stresses and strains are assigned negative values. Principal stresses (P1 tensile; P3 compressive), shear stress in the sagittal (here XY) 2D plane, normal X, normal Y and sagittal XY shear strain were recorded (the software does not calculate principal strains). Principal stresses record peak compressive and tensile stresses when shear stress equals zero. Peak tensile, compressive and shear stresses and strains were recorded and treated as an indicator of skull ‘strength’: higher peak stresses mean that less force is needed to induce yielding, therefore the skull is weaker. Regardless of bite force magnitude (moment-arm versus ‘tooth-size’ forces), nearly identical patterns of stress and strain were produced in models of the same geometry (although absolute magnitudes differ). It can be assumed that the stress patterns figured here apply to either biting regime.

(a) Stress in the fused-skull finite element model during biting and tearing

Stress patterns in the vertical biting model (mimicking the ‘puncture’ phase of feeding) suggest that during biting, compressive stresses arc posterodorsally from the biting teeth through the maxilla and into the nasals and lacrimals ( figure 3 a ). Stress vectors trace this curvature then become longitudinally orientated in the posterior region of the nasals and dorsal body of the postorbital ( figure 3 a ). Peak tensile stresses are orientated longitudinally within the jugal and posterior maxilla, ventral to the lower temporal fenestra, orbit and antorbital fenestra ( figure 3 b ). Tension follows the ventral rim of the antorbital fenestra, leaving the main body of the maxilla dorsal to the tooth row relatively untensed ( figure 3 b ). Peak shear occurs in the nasals dorsal to the central antorbital fenestra and dorsal to the orbit ( figure 3 c ).

When the biting simulation is altered to reflect pulling and tearing (hereafter known as the ‘tearing’ model), tensile vectors lose their anterodorsal component and trace the ventral edge of the skull ( figure 3 e ). The largest maxillary teeth are subject to bending stress: the posterior tooth edge is tensed while the anterior edge compresses along its curvature ( figure 3 d, e ). Compressive vectors are less obvious in the maxilla but longitudinally orientated compression is maintained in the dorsal maxilla, nasal and lacrimal ( figure 3 d ). Large shear stresses are still observed in the nasals as during vertical biting, and the teeth are sheared also ( figure 3 f ). Considering that the angle of bite force shifts by 90° from biting to tearing, stress distribution and orientation are surprisingly similar in both sets of models. There are, however, noticeable differences in stress–strain magnitude between the two loading conditions ( table 1 ).

(b) Predicting the effect of introducing cranial mobility from solid finite element models

(i) Maxilla–jugal suture

This suture is located at the point of peak tensile stress in the biting skull model, and at a region of high magnitude (but not peak) tension in the tearing skull model ( figure 3 b, e ). Tensile vectors are oriented along the predicted axis of suture movement (slightly more so in biting than tearing: compare figures 2 b and 3 b, e ) and it is predicted that the introduction of suture mobility will act to reduce regional tensile stress, although whether the skull will be weaker or stronger is unclear. Small compressive vectors act perpendicularly to the axis of movement in the biting skull (not shown) and may operate to maintain contact of opposing joint surfaces.

(ii) Postorbital–jugal suture

Low-magnitude compressive vectors act along the long axis of the postorbital–jugal strut during biting and tearing, and tensile stresses are absent ( figure 3 a–d). This pattern is not congruent with the predicted axis of postorbital–jugal suture movement ( figure 2 a ). It would therefore be predicted that mobilizing the postorbitaljugal suture should have a negligible effect upon stress distribution and overall strength of the skull under both biting regimes.

(c) The effect of introducing sutures into a finite element model

As predicted, introducing a mobile postorbital–jugal suture into a FE-skull model has no notable effect on stress distribution and magnitude during biting and tearing ( table 1 and compare figure 4 a, b with figure 3 a, b and figure 4 e, f with figure 3 d, e ). Apart from a loss of compression in the postorbital bar (compare figure 3 a, d with figure 4 a, e ) and marginal alterations to shear stress (see electronic Appendices A and B) in the mobile postorbitaljugal model, the stress environment and peak stresses and strain are practically identical to that of the fused skull.

Introducing a mobile contact at the maxilla–jugal suture removes tensile and shear stresses along the ventral region of the skull model. Peak tensile, compressive and shear stresses are instead concentrated in the posterior portion of the nasals and in the lacrimal dorsal to the antorbital fenestra (compare figure 3 a, b with figure 4 c, d and figure 3 d, e with figure 4 g, h ; see electronic Appendices A and B for shear plots). Stress distribution is comparable during biting and tearing (compare biting figure 4 c, d to tearing figure 4 g, h ). Dorsal to the antorbital fenestra, the skull experiences bending stresses as the lacrimal and possibly the posterior maxilla experience tension as the nasals are compressed ( figure 4 c, d, g, h ). Although opening the max-illa–jugal suture has removed large tensile stresses from the ventral skull, peak stresses have been concentrated dorsal to the antorbital fenestra at magnitudes of 7 to 11 times greater than fused model peak stress–strain values ( table 1 ). The dominant effect of the maxilla–jugal suture is such that the introduction of a second mobile joint at the postorbital–jugal contact has no modifying effect on mechanical performance and cranial stress patterns (see electronic Appendix A).

4. DISCUSSION

Stress–strain distribution and orientation are remarkably similar during simulations of both biting and tearing. Morphological features that resist biting loads are used equally in the resistance of tearing forces, meaning that the skull appears to be equally well adapted for the ‘puncture’ and ‘pull’ components of the proposed feeding strategy. Fused-skull models and those with a mobile postorbital–jugal suture are characterized by ventral tension and posterodorsally arcing compression from the tooth row to the skull roof, whereas models with a mobile maxilla–jugal suture experience bending stress in the roof of the snout, dorsal to the antorbital fenestra. Tensile and compressive patterns appear similar, but not identical, to those observed in a three-dimensional (3D) Allosaurus fragilis FEM during bilateral bite loading ( Rayfield et al. 2001; figure 3 ).

(a) Rostral stress transmission

It has been suggested that T. rex cranial suture morphology dictates that biting-induced compressive stresses pass directly from the maxilla to the nasals and bypass the maxilla–lacrimal contact ( Hurum & Sabath 2003). FEMs confirm that compressive stresses do bypass the lacrimal when the maxilla–jugal suture is mobile ( figure 4 c, g ). The maxilla–lacrimal contact is subject to large tensile bending stresses instead ( figure 4 d, h ). The complex interlacing morphology of the maxilla–nasal suture is consistent with the efficient accommodation of compressive strain and shock-absorption ( Jaslow 1990) and the groove-like morphology of the maxilla–lacrimal contact suggests an adaptation to accommodate tensile strain across this suture. Nevertheless, when the maxilla–jugal suture is immobilized in the fused skull models, high-magnitude compressive stresses do pass directly from maxilla to lacrimal ( figure 3 a, d and 4 a, e ). This observation questions the distinctions drawn between the skulls of T. rex and Tarbosaurus baatar based upon compressive stress transmission ( Hurum & Sabath 2003).

(b) Nasal robustness and rugosities

Tyrannosaurid nasals are extremely rugose dorsally, and fused along the majority of their length, while the postorbitals display a dorsal, laterally expanded, thickened boss with a roughened surface ( figures 1 a and 2 a ‘po’). In all FEMs, peak compressive and shear stresses are concentrated in the nasals and dorsal portion of the postorbital, particularly when the maxilla–jugal suture is mobilized. The morphology of these rugose cranial bones suggests that they are optimized to withstand the type of compressive, shearing and bending stresses predicted by the FEM. As fused nasals are found in all tyrannosaurids and the tyrannosauroid Eotyrannus lengi ( Hutt et al. 2001) perhaps we should expect to see similar patterns of cranial stress distribution in all members of the Tyrannosauroidea. In marked contrast, peak compressive and shear stresses accumulate in the fronto-parietal region rather than the nasals in biting A. fragilis FEMs ( Rayfield 2001; Rayfield et al. 2001). As predicted by the FE-stress patterns, the frontals and parietals are fused or strongly sutured and thickened, and although the lateral borders of A. fragilis nasals are rugose, medially they are smooth elements meeting at a midline butt-joint that is often patent.

Nasal robustness and dorsal protuberances become more pronounced throughout T. rex ontogeny ( Carr 1999) and this may be consistent with resisting greater bite forces in more mature individuals, if bite force scales with positive allometry to body mass and length as seen in the American alligator Alligator mississippiensis ( Erickson et al. 2003). In an unusual example of less robust nasals (FMNH PR2081), prominent nasal protuberances are still observed dorsal to the antorbital fenestra ( Brochu 2003), in the region predicted by the FEMs.

(c) Cantilever bending and lacrimal morphology

Patterns of dorsal compression and ventral tension are consistent with the nasal region of the skull bending as a cantilever beam during biting. Even so, the presence of stress in the lacrimal and postorbital bars demonstrates that the skull does not act as a simple beam in the manner suggested by Molnar (2000), because the postulated neutral axis of bending in the region occupied by the interfenestral bars does in fact experience stress. Furthermore, modelled stress patterns in the lacrimal can be correlated with bony morphology as the axis of biting-induced compressive stress lies along a thin but medially prominent ridge of bone in the T. rex lacrimal (e.g. figures 3 a and 4 a ). When the postorbital–jugal suture is open during tearing, this ridge withstands tensile stress instead ( figure 4 f ).

(d) Tensile resistance

According to FEMs, the postorbital–jugal suture did not play an active role in cranial stress accommodation, despite the sliding nature of the joint. The suture may not be mechano-functionally adapted or the position of model constraints may be affecting this result, and it should be investigated in future models. By contrast, FEMs suggest that the maxilla–jugal suture of T. rex was adapted to resist biting- and tearing-induced tensile strain in the ventral skull. Regardless of how mobile the sutures were in life, even minor adjustments in articulation would have served to protect bony tissue from damaging strains. The simple morphology of the maxilla–jugal suture is consistent with the observation that decreasing interdigitation and lack of fusion are associated with the presence of tensile strains at mammalian sutures ( Rafferty & Herring 1999).

As a consequence of removing tension in the ventral skull, stresses and strains are directed elsewhere. In the case of the mobile maxilla–jugal model, stresses an order of magnitude greater than those generated in the fused model are experienced in the nasals, maxilla and lacrimal. During actual dynamic loading in υiυo, sutural ligaments could act as shock-absorbers, absorbing tensile strain energy and reducing the magnitude of stress and strain in the dorsal skull, so increasing the adaptive significance of the suture. But it still appears fair to say that, as safety factors appear constant across taxa of all sizes, although higher in crocodilians than mammals and birds ( Biewener 1982; Thomason & Russell 1986; Blob & Biewener 1999), the introduction of a mobile maxilla–jugal suture effectively ‘weakens’ the skull model, such that lower maximum bite forces can be tolerated, to maintain a constant ratio between stress generated during everyday use and peak yield stress (i.e. the safety factor). Although the maxilla–jugal suture is locally adapted to stress resistance, there is an overall functional cost of introducing this suture in terms of reduced skull strength. Fused skull models and those with mobility at the postorbital–jugal suture are ‘stronger’ and can tolerate higher maximum bite forces while maintaining a similar margin of safety.

The FEMs presented here are obviously crude representations of skull geometry and suture mobility. Strain-absorbing soft tissues are absent and loads are not transmitted across the suture as they are in υiυo and in υitro ( Buckland-Wright 1978; Thomason et al. 2001). Nevertheless, generating testable predictions and correlation of stress patterns to cranial morphology is possible using these simple models. Factors such as investigating the performance of a 3D model, altering the position of constraints and incorporating soft tissues at tooth sockets and further sutural contacts will all advance our understanding of T. rex cranial mechanical behaviour.

5. CONCLUSION

The cranium of T. rex appears equally well adapted to resist biting and tearing loading. This suggests that the puncture–pull feeding strategy inferred from toothmarked bones is consistent with the mechanical construction and performance of the skull. Stress patterns predicted by FEMs are consistent with the bony morphology of the skull and a number of form–function adaptations can be identified.

(i) The robust nasals, positioned along the dorsal edge of the rostrum, act to resist compressive and shear stresses. This raises questions as to the evolution of tyrannosauroid nasal robusticity in relation to feeding behaviour: did the evolution of robusticity permit a shift in feeding strategy or did a novel strategy arise in which robusticity was advantageous?

(ii) The lacrimal is constructed to resist a complex suite of stresses found during simulated biting and tearing.

(iii) The maxilla–nasal contact acts to dissipate biting loads as previously suggested.

(iv) The maxilla–jugal suture appears to be adapted to resist tension in the ventral skull although at a cost of reduced cranial strength and capacity.

Sutural fusion appears to be controlled by an interplay of genetic and epigenetic factors ( Herring 2000). The detrimental weakening effect of loosening the maxilla–jugal suture raises the possibility that mobility of sutures evolved in a correlated manner as an adaptive response to resist potentially damaging stresses generated during particular feeding styles, although the behaviour of the post-orbital–jugal suture challenges the idea that all sutures are functionally adaptive.

Using stress vectors generated in fused cranial models it is possible to predict the localized mechanical effect of introducing sutural mobility and the possible functional role and adaptive significance of the suture concerned. There is considerable potential for the use of FEA in the elucidation of patterns of cranial evolution, including the development of intracranial mobility within and across groups. However, steps towards modelling of soft tissues that are also integral to the behaviour of the cranium must be taken to achieve a more complete understanding of such morpho-functional evolutionary events.

Figures

Figure 1. Tyrannosaurus rex skull and FEM. (a) Skull of BHM 3033, left lateral view; and (b) 2D FE-mesh of BHM 3033 depicting skull as ‘fused’ without mobile sutures. Grey areas indicate surfaces constrained from moving in all translatory directions, arrows indicate direction of bite force applied to all teeth, either vertical or horizontal ‘tearing’. Abbreviations: aof, antorbital fenestra; en, external naris; j, jugal; l, lacrimal; ltf, lower temporal fenestra; m, maxilla, n, nasals; or, orbit; p, premaxilla; po, postorbital; pt, pterygoid; q, quadrate; qj, quadratojugal; sq, squamosal. Scale bar 10 cm.

Figure 2. Sutural morphology and mobility. (a) Postorbital–jugal suture in Tyrannosaurus rex; (b) maxilla–jugal suture in T. rex; (c) 2D FEM of T. rex skull with mobile postorbital–jugal contact; and (d) 2D FEM of T. rex skull with mobile maxillajugal contact. Double-headed arrows indicate direction of slight adjustive movement at suture. Single-headed arrows indicate location of ‘suture’ in FE-mesh. Illustrations after BHM 3033. Grey areas and abbreviations as defined in figure 1; pal, palatine.

Figure 3. Stress in the fused FE Tyrannosaurus rex skull model generated by vertical biting (left column) or tearing (right column). (a) Principal stress 3 [P3], compressive stress; (b) P1 tensile stress; (c) shear stress; (d) P3 compressive stress; (e) P1 tensile stress; and (f) shear stress. Divergent arrows indicate orientation of tensile stress trajectories; convergent arrows indicate orientation of compressive stress trajectories. Units are Pa or Nm‾2. See electronic Appendix C for strain plots.

Figure 4. Stress in the mobile FE Tyrannosaurus rex skull models generated by vertical biting (left column) or tearing (right column). (a,b,e, f) have a mobile postorbital–jugal suture; (c,d,g,h) have a mobile maxilla–jugal suture. (a) Principal stress 3 [P3], compressive stress; (b) P1 tensile stress; (c) P3 compressive stress; (d) P1 tensile stress; (e) P3 compressive stress; (f) P1 tensile stress; (g) P3 compressive stress; and (h) P1 tensile stress. Divergent arrows indicate orientation of tensile stress trajectories; convergent arrows indicate orientation of compressive stress trajectories. Units are Pa or Nm–2. See electronic Appendices B and C for shear stress and strain plots.

Tables

Table 1. Comparison of peak stress and strain values: owing to the simplistic nature of model, regard values as relative rather than absolute. (Stress values are megapascals (MPa); strain values are microstrain (με); X or Y refers to direction of peak strain.)

		peak tensile stress (P1)	peak compressive stress (P3)	peak shear stress	peak tensile strain	peak compressive strain	peak XY shear strain
vertical biting	fused model	11.4	‾ 12.1	4.6	1100 (X)	‾ 1100 (X)	1300
	post.-jugal kinesis	10.2	‾ 14.1	5.1	1160 (X)	‾ 1300 (X)	1620
	max.-jugal kinesis	85.2	‾ 117.0	43.2	12400 (X)	‾ 11500 (X)	12400
	double kinesis	85.2	‾ 117.0	43.2	12400 (X)	‾ 11500 (X)	12400
tearing biting	fused model	16.8	‾ 19.4	7.2	2080 (Y)	‾ 1830 (Y)	2170
	post.-jugal kinesis	13.9	‾ 19.5	7.3	2080 (Y)	‾ 1820 (Y)	2170
	max.-jugal kinesis	120.8	‾ 156.4	54.3	16190 (X)	‾ 14320 (X)	15810
	double kinesis	120.8	‾ 156.4	54.3	16200 (X)	‾ 14330 (X)	15810